Tones

In Beginner Music Theory For Guitar pt1 we learned a little about sound. Hopefully you have looked into those ideas a little bit further on your own. We know that we can hear frequencies between 20hz and 20khz. Any constant, sustained vibration between those two frequencies will produce a tone, and tones are what we make music out of.

Notes

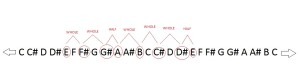
Sometime in the past, it was decided that a “A” Note would be a tone at the frequency of 440hz. Now, an interesting thing happens if you play a tone at the frequency of 880hz (440hz x 2). The two tones are extremely similar (except 880hz has a higher pitch), so much so that if they are played together they sound like one note. We call this an Octave, and it happens every time you double the frequency of a tone. So, we call the tone at 880hz an “A” as well, and the tone at 1760hz is an “A”, and so on. It was decided to divide the frequencies between 440hz and 880hz (an Octave) into 12 equal “half steps” (Notes) and label them as follows.
A, A#, B, C, C#, D, D#, E, F, F#, G, G#.
A half step is one note up or or one note down from another note. B is a half step down from C and a half step up from A#. A whole step is two half steps. D is a whole step up from C and a whole step down from E.

Scales

It was discovered that, when writing music, all of the notes did not always sound good together and songs worked much better when they were in a defined Key (similar to a color scheme). You establish a Key by using a Scale, which is a specific set of the twelve notes. There are many recognized scales, but by far the most popular one is the Major Scale. Almost all of the music you know is created using it. We create a Major scale using a simple formula that we apply to the 12 notes. The formula for a Major scale is:
Whole Tone – Whole Tone – Half Tone – Whole Tone – Whole Tone – Whole Tone – Half Tone.
This formula can be applied to any of the 12 notes to get the Major scale for that note.

A MAJOR

So, to make a A MAJOR scale we start with an A note and ADD the formula to it. A, whole tone = B, whole tone = C#, half tone = D, whole tone = E, whole tone = F#, whole tone = G#, half tone = A.
A MAJOR = A,B,C#,D,E,F#,G#,A. Notice that we ended on the same note we began with.

E MAJOR

To make a E MAJOR scale we start with an E note and ADD the formula to it. E, whole tone = F#, whole tone = G#, half tone = A, whole tone = B, whole tone = C#, whole tone = D#, half tone = E.
E MAJOR = E,F#,G#,A,B,C#,D#,E. Notice that we ended on the same note we began with once again.

Now we have learned how to make the Major scale out of the 12 tones. Next we will learn how to make Chords.

PART 3